First level

The degree and properties. Exhaustive guide (2019)

Why are you needed? Where will they come to you? Why do you need to spend time on their study?

To find out all about the degrees, what they need for what they need how to use their knowledge in everyday life read this article.

And, of course, the knowledge of degrees will bring you closer to the successful surrender of OGE or the EGE and to enter the university of your dreams.

Let "S GO ... (drove!)

Important remark! If instead of formulas you see abracadabra, clean the cache. To do this, click Ctrl + F5 (on Windows) or CMD + R (on Mac).

FIRST LEVEL

The exercise is the same mathematical operation as addition, subtraction, multiplication or division.

Now I will explain all the human language on very simple examples. Pay attention. Examples of elementary, but explaining important things.

Let's start with addition.

There is nothing to explain here. You all know everything: we are eight people. Everyone has two bottles of cola. How much is the cola? Right - 16 bottles.

Now multiplication.

The same example with a cola can be recorded differently :. Mathematics - People cunning and lazy. They first notice some patterns, and then invent the way how to "count" them faster. In our case, they noticed that each of the eight people had the same number of cola bottles and came up with a reception called multiplication. Agree, it is considered easier and faster than.

So, to read faster, easier and without mistakes, you just need to remember table multiplication. Of course, you can do everything more slowly, harder and mistakes! But…

Here is the multiplication table. Repeat.

And the other, more beautiful:

And what else clear receptions Increased maritime mathematics? Right - erection.

Erection

If you need to multiply the number for yourself five times, then mathematics say that you need to build this number in the fifth degree. For example, . Mathematics remember that two in the fifth degree is. And they solve such tasks in the mind - faster, easier and without errors.

For this you need only remember what is highlighted in color in the table of degrees of numbers. Believe it, it will greatly facilitate your life.

By the way, why the second degree is called square numbers, and the third - cuba? What does it mean? Highly good question. Now there will be to you and squares, and Cuba.

Example from life number 1

Let's start with a square or from a second degree of number.

Imagine a square pool of meter size on a meter. The pool is on your dacha. Heat and really want to swim. But ... Pool without the bottom! You need to store the bottom of the pool tiles. How much do you need tiles? In order to determine this, you need to find out the area of \u200b\u200bthe bottom of the pool.

You can simply calculate, with a finger, that the bottom of the pool consists of a meter cubes per meter. If you have a meter tile for meter, you will need to pieces. It's easy ... But where did you see such a tile? The tile is more likely to see for see and then "finger to count" torture. Then you have to multiply. So, on one side of the bottom of the pool, we fit tiles (pieces) and on the other too tiles. Multiplying on, you will get tiles ().

Did you notice that in order to determine the area of \u200b\u200bthe bottom of the pool, did we multiply the same number by yourself? What does it mean? This is multiplied by the same number, we can take advantage of the "erection of the extermination". (Of course, when you have only two numbers, multiply them or raise them into the degree. But if you have a lot of them, it is much easier to raise them in terms of calculations, too much less. For the exam, it is very important).
So thirty to the second degree will (). Or we can say that thirty in the square will be. In other words, the second degree of number can always be represented as a square. And on the contrary, if you see a square - it is always the second degree of some number. Square is the image of a second degree number.

Example from life number 2

Here is the task, count how many squares on a chessboard with a square of the number ... on one side of the cells and on the other too. To calculate their quantity, you need to multiply eight or ... If you note that the chessboard is a square of the side, then you can build eight per square. It turns out cells. () So?

Example from life number 3

Now a cube or the third degree of number. The same pool. But now you need to know how much water will have to fill in this pool. You need to count the volume. (Volume and liquid, by the way, are measured in cubic meters. Suddenly, right?) Draw a pool: bottom of the meter size and a depth of meter and try to count how much cubes meter size per meter will enter your pool.

Right show your finger and count! Once, two, three, four ... twenty two, twenty three ... how much did it happen? Did not come down? Difficult to count your finger? So that! Take an example with mathematicians. They are lazy, therefore noticed that to calculate the volume of the pool, it is necessary to multiply each other in length, width and height. In our case, the volume of the pool will be equal to cubes ... it is easier for the truth?

And now imagine, as far as Mathematics are lazy and cunning, if they are simplified. Brought all to one action. They noticed that the length, width and height is equal to and that the same number varnims itself on itself ... And what does this mean? This means that you can take advantage of the degree. So, what did you think with your finger, they do in one action: three in Cuba is equal. This is written so :.

It remains only remember Table degrees. If you are, of course, the same lazy and cunning as mathematics. If you like to work a lot and make mistakes - you can continue to count your finger.

Well, to finally convince you that the degrees came up with Lodii and cunnies to solve their life problems, and not to create problems you, here's another couple of examples from life.

Example from life number 4

You have a million rubles. At the beginning of each year you earn every million another million. That is, every million will double at the beginning of each year. How much money will you have in the years? If you are sitting now and "you think your finger", then you are a very hardworking person and .. stupid. But most likely you will answer in a couple of seconds, because you are smart! So, in the first year - two multiplied two ... in the second year - what happened, another two, on the third year ... Stop! You noticed that the number multiplies itself. So, two in the fifth degree - a million! And now imagine that you have a competition and these million will receive the one who will find faster ... It is worth remembering the degree of numbers, what do you think?

Example from life number 5

You have a million. At the beginning of each year you earn each million two more. Great truth? Every million triples. How much money will you have after a year? Let's count. The first year is to multiply on, then the result is still on ... already boring, because you have already understood everything: three is multiplied by itself. Therefore, the fourth degree is equal to a million. It is only necessary to remember that three in the fourth degree is or.

Now you know that with the help of the erection of the number, you will greatly facilitate your life. Let's look next to what you can do with the degrees and what you need to know about them.

Terms and concepts ... so as not to get confused

So, for starters, let's define the concepts. What do you think, what is the indicator of the degree? It is very simple - this is the number that is "at the top" of the degree of number. Not scientifically, but it is clear and easy to remember ...

Well, at the same time that such a foundation degree? Even easier - this is the number that is below, at the base.

Here is a drawing for loyalty.

Well, B. generalTo summarize and better remember ... The degree with the foundation "" and the indicator "" is read as "to degree" and is written as follows:

The degree of number with a natural indicator

You already probably guessed: because the indicator is natural number. Yes, but what is natural number? Elementary! Natural These are the numbers that are used in the account when listing items: one, two, three ... We, when we consider items, do not say: "Minus five", "minus six", "minus seven". We also do not say: "one third", or "zero of whole, five tenths." These are not natural numbers. And what these numbers do you think?

Numbers like "minus five", "minus six", "minus seven" belong to whole numbers. In general, to whole numbers include all natural numbers, the numbers are opposite to natural (that is, taken with a minus sign), and the number. Zero understand easily - this is when nothing. And what do they mean negative ("minus") numbers? But they were invented primarily to designate debts: if you have a balance on the phone number, it means that you should operator rubles.

All sorts of fractions are rational numbers. How did they arise, what do you think? Very simple. Several thousand years ago, our ancestors found that they lack natural numbers to measure long, weight, square, etc. And they invented rational numbers... I wonder if it's true?

There are also irrational numbers. What is this number? If short, then infinite decimal. For example, if the circumference length is divided into its diameter, then the irrational number will be.

Summary:

We define the concept of degree, the indicator of which is a natural number (i.e., a whole and positive).

1. Any number to the first degree equally to itself:
2. Evaluate the number in the square - it means to multiply it by itself:
3. Evaluate the number in the cube - it means to multiply it by itself three times:

Definition. Evaluate the number in a natural degree - it means to multiply the number of all time for yourself:
.

Properties of degrees

Where did these properties come from? I will show you now.

Let's see: what is and ?

A-priory:

How many multipliers are here?

Very simple: we completed multipliers to multipliers, it turned out the factors.

But by definition, this is the degree of a number with an indicator, that is, that, that it was necessary to prove.

Example: Simplify the expression.

Decision:

Example: Simplify the expression.

Decision: It is important to notice that in our rule before Must be the same foundation!
Therefore, we combine degrees with the basis, but remains a separate multiplier:

only for the work of degrees!

In no case can not write that.

2. That is The degree of number

Just as with the previous property, we turn to the definition of the degree:

It turns out that the expression is multiplied by itself once, that is, according to the definition, this is, there is a number of number:

In fact, this can be called "the indicator for brackets". But never can do it in the amount:

Recall the formula of abbreviated multiplication: how many times did we want to write?

But it is incorrect, because.

Negative

Up to this point, we only discussed what the indicator should be.

But what should be the basis?

In the degrees of S. natural indicator The base can be any number. And the truth, we can multiply each other any numbers, whether they are positive, negative, or even.

Let's think about what signs ("or" ") will have the degrees of positive and negative numbers?

For example, a positive or negative number? BUT? ? With the first, everything is clear: how many positive numbers we are not multiplied by each other, the result will be positive.

But with negative a little more interesting. After all, we remember a simple rule of grade 6: "Minus for minus gives a plus." That is, or. But if we multiply on, it will work out.

Determine independently, what sign the following expressions will have:

1)	2)	3)
4)	5)	6)

Cope?

Here are the answers: in the first four examples, I hope everything is understandable? Just look at the base and indicator, and apply the appropriate rule.

1) ; 2) ; 3) ; 4) ; 5) ; 6) .

In example 5), everything is also not as scary, as it seems: it doesn't matter what is equal to the base - the degree is even, which means that the result will always be positive.

Well, with the exception of the case when the base is zero. The reason is not equal? Obviously no, because (because).

Example 6) is no longer so simple!

6 Examples for Training

Solutions of 6 examples

If you do not pay attention to the eighth degree, what do we see here? Remember the Grade 7 program. So, remembered? This is a formula for abbreviated multiplication, namely - the difference of squares! We get:

Carefully look at the denominator. He is very similar to one of the multipliers of the numerator, but what's wrong? Not the procedure of the terms. If they would change them in places, it would be possible to apply the rule.

But how to do that? It turns out very easy: the even degree of denominator helps us.

Magically, the components changed in places. This "phenomenon" is applicable for any expression to an even degree: we can freely change signs in brackets.

But it is important to remember: all signs are changing at the same time.!

Let's go back for example:

And again the formula:

Integer We call natural numbers opposite to them (that is, taken with the sign "") and the number.

whole positive number, And it does not differ from natural, then everything looks exactly as in the previous section.

And now let's consider new cases. Let's start with an indicator equal to.

Any number to zero equal to one:

As always, we will ask me: why is it so?

Consider any degree with the basis. Take, for example, and domineering on:

So, we multiplied the number on, and got the same as it was. And for what number must be multiplied so that nothing has changed? That's right on. So.

We can do the same with an arbitrary number:

Repeat the rule:

Any number to zero equal to one.

But from many rules there are exceptions. And here it is also there is a number (as a base).

On the one hand, it should be equal to any extent - how much zero itself is neither multiplied, still get zero, it is clear. But on the other hand, like any number to zero degree, should be equal. So what's the truth? Mathematics decided not to bind and refused to erect zero to zero. That is, now we can not only be divided into zero, but also to build it to zero.

Let's go further. In addition to natural numbers and numbers include negative numbers. To understand what a negative degree, we will do as last time: Domingly some normal number on the same to a negative degree:

From here it is already easy to express the desired:

Now we spread the resulting rule to an arbitrary degree:

So, we formulate the rule:

The number is a negative degree back to the same number to a positive degree. But at the same time the base can not be zero: (Because it is impossible to divide).

Let's summarize:

I. The expression is not defined in the case. If, then.

II. Any number to zero is equal to one :.

III. A number that is not equal to zero, to a negative degree back to the same number to a positive degree :.

Tasks for self solutions:

Well, as usual, examples for self solutions:

Task analysis for self solutions:

I know, I know, the numbers are terrible, but the exam should be ready for everything! Share these examples or scatter their decision, if I could not decide and you will learn to easily cope with them on the exam!

Continue expanding the circle of numbers, "suitable" as an indicator of the degree.

Now consider rational numbers. What numbers are called rational?

Answer: All that can be represented in the form of fractions, where and - integers, and.

To understand what is "Freight degree", Consider the fraction:

Erected both parts of the equation to the degree:

Now remember the rule about "Degree to degree":

What number should be taken to the degree to get?

This formulation is the definition of root degree.

Let me remind you: the root of the number () is called the number that is equal in the extermination.

That is, the root degree is an operation, reverse the exercise into the degree :.

Turns out that. Obviously this private case You can expand :.

Now add a numerator: what is? The answer is easy to get with the help of the "degree to degree" rule:

But can the reason be any number? After all, the root can not be extracted from all numbers.

No one!

Remember the rule: any number erected into an even degree is the number positive. That is, to extract the roots of an even degree from negative numbers it is impossible!

This means that it is impossible to build such numbers into a fractional degree with an even denominator, that is, the expression does not make sense.

What about expression?

But there is a problem.

The number can be represented in the form of DRGIH, reduced fractions, for example, or.

And it turns out that there is, but does not exist, but it's just two different records of the same number.

Or another example: once, then you can write. But it is worthwhile to write to us in a different way, and again we get a nuisance: (that is, they received a completely different result!).

To avoid similar paradoxes, we consider only a positive foundation of degree with fractional indicator.

So, if:

• - natural number;
• - integer;

Examples:

The degrees with the rational indicator are very useful for converting expressions with roots, for example:

5 examples for training

Analysis of 5 examples for training

Well, now - the most difficult. Now we will understand irrational.

All the rules and properties of degrees here are exactly the same as for a degree with a rational indicator, with the exception

After all, by definition, irrational numbers are numbers that cannot be represented in the form of a fraction, where and - integers (that is, irrational numbers are all valid numbers except rational).

When studying degrees with natural, whole and rational indicator, we each time constituted a certain "image", "analogy", or a description in more familiar terms.

For example, a natural figure is a number, several times multiplied by itself;

...zero - this is how the number multiplied by itself once, that is, it has not yet begun to multiply, it means that the number itself has not even appeared - therefore the result is only a certain "billet number", namely the number;

...degree with a whole negative indicator "It seemed to have occurred a certain" reverse process ", that is, the number was not multiplied by itself, but Deli.

By the way, in science is often used with a complex indicator, that is, the indicator is not even a valid number.

But at school we do not think about such difficulties, you will have the opportunity to comprehend these new concepts at the Institute.

Where we are sure you will do! (If you learn to solve such examples :))

For example:

Solim yourself:

Debris:

1. Let's start with the usual rules for the exercise rules for us:

Now look at the indicator. Doesn't he remind you of anything? Remember the formula of abbreviated multiplication. Square differences:

In this case,

Turns out that:

Answer: .

2. We bring the fraction in the indicators of degrees to the same form: either both decimal or both ordinary. We obtain, for example:

Answer: 16.

3. Nothing special, we use the usual properties of degrees:

ADVANCED LEVEL

Determination of degree

The degree is called the expression of the form: where:

• — degree basis;
• - Indicator.

The degree with the natural indicator (n \u003d 1, 2, 3, ...)

Build a natural degree n - it means multiplying the number for yourself once:

The degree with the integer (0, ± 1, ± 2, ...)

If an indicator of the degree is software positive number:

Construction in zero degree:

The expression is indefinite, because, on the one hand, to any extent, it is, and on the other - any number of in degree is.

If an indicator of the degree is a whole negative number:

(Because it is impossible to divide).

Once again about zeros: the expression is not defined in the case. If, then.

Examples:

Rational

• - natural number;
• - integer;

Examples:

Properties of degrees

To make it easier to solve problems, let's try to understand: where did these properties come from? We prove them.

Let's see: What is what?

A-priory:

So, in the right part of this expression, such a work is obtained:

But by definition, this is the degree of a number with an indicator, that is:

Q.E.D.

Example : Simplify the expression.

Decision : .

Example : Simplify the expression.

Decision : It is important to notice that in our rule beforethere must be the same bases. Therefore, we combine degrees with the basis, but remains a separate multiplier:

Another important note: this is a rule - only for the work of degrees!

In no case to the nerve to write that.

Just as with the previous property, we turn to the definition of the degree:

We regroup this work like this:

It turns out that the expression is multiplied by itself once, that is, according to the definition, this is - by the degree of number:

In fact, this can be called "the indicator for brackets". But never can do this in the amount:!

Recall the formula of abbreviated multiplication: how many times did we want to write? But it is incorrect, because.

Degree with a negative basis.

Up to this point, we only discussed what should be indicator degree. But what should be the basis? In the degrees of S. natural indicator The base can be any number .

And the truth, we can multiply each other any numbers, whether they are positive, negative, or even. Let's think about what signs ("or" ") will have the degrees of positive and negative numbers?

For example, a positive or negative number? BUT? ?

With the first, everything is clear: how many positive numbers we are not multiplied by each other, the result will be positive.

But with negative a little more interesting. After all, we remember a simple rule of grade 6: "Minus for minus gives a plus." That is, or. But if we will multiply on (), it turns out.

And so to infinity: each time the next multiplication will change the sign. You can formulate such simple rules:

1. even degree - number positive.
2. A negative numbererected by odd degree - number negative.
3. A positive number to either degree is the number positive.
4. Zero to any degree is zero.

Determine independently, what sign the following expressions will have:

1.	2.	3.
4.	5.	6.

Cope? Here are the answers:

1) ; 2) ; 3) ; 4) ; 5) ; 6) .

In the first four examples, I hope everything is clear? Just look at the base and indicator, and apply the appropriate rule.

In example 5), everything is also not as scary, as it seems: it doesn't matter what is equal to the base - the degree is even, which means that the result will always be positive. Well, with the exception of the case when the base is zero. The reason is not equal? Obviously no, because (because).

Example 6) is no longer so simple. Here you need to know that less: or? If you remember that it becomes clear that, and therefore, the base is less than zero. That is, we apply the rule 2: the result will be negative.

And again we use the degree of degree:

All as usual - write down the definition of degrees and, divide them to each other, divide on the pairs and get:

Before you disassemble the last rule, we solve several examples.

Calculated expressions:

Solutions :

If you do not pay attention to the eighth degree, what do we see here? Remember the Grade 7 program. So, remembered? This is a formula for abbreviated multiplication, namely - the difference of squares!

We get:

Carefully look at the denominator. He is very similar to one of the multipliers of the numerator, but what's wrong? Not the procedure of the terms. If they were swapped in places, it would be possible to apply the rule 3. But how to do it? It turns out very easy: the even degree of denominator helps us.

If you draw it on, nothing will change, right? But now it turns out the following:

Magically, the components changed in places. This "phenomenon" is applicable for any expression to an even degree: we can freely change signs in brackets. But it is important to remember: all signs are changing at the same time!You can not replace on, changing only one disagreeable minus!

Let's go back for example:

And again the formula:

So now the last rule:

How will we prove? Of course, as usual: I will reveal the concept of degree and simplifies:

Well, now I will reveal brackets. How much will the letters get? Once on multipliers - what does it remind? It is nothing but the definition of the operation multiplication: In total there were factors. That is, it is, by definition, the degree of number with the indicator:

Example:

Irrational

In addition to information about degrees for the average level, we will analyze the degree with the irrational indicator. All the rules and properties of degrees here are exactly the same as for a degree with a rational indicator, with the exception - after all, by definition, irrational numbers are numbers that cannot be submitted in the form of a fraction, where - the integers (i.e., irrational numbers are All valid numbers besides rational).

When studying degrees with natural, whole and rational indicator, we each time constituted a certain "image", "analogy", or a description in more familiar terms. For example, a natural figure is a number, several times multiplied by itself; The number in zero degree is somehow the number multiplied by itself once, that is, it has not yet begun to multiply, it means that the number itself has not even appeared - therefore, only a certain "billet", namely, is the result; The degree with a whole negative indicator is as if a certain "reverse process" occurred, that is, the number was not multiplied by itself, but divided.

Imagine the degree with an irrational indicator is extremely difficult (just as it is difficult to submit a 4-dimensional space). It is rather a purely mathematical object that mathematics created to expand the concept of degree to the entire space of numbers.

By the way, in science is often used with a complex indicator, that is, the indicator is not even a valid number. But at school we do not think about such difficulties, you will have the opportunity to comprehend these new concepts at the Institute.

So what do we do if we see an irrational rate? We are trying to get rid of it with all the might! :)

For example:

Solim yourself:

1)

2)

3)

Answers:

1. We remember the formula the difference of squares. Answer:.
2. We give the fraction to the same form: either both decimal, or both ordinary. We get, for example:.
3. Nothing special, we use the usual properties of degrees:

Summary of section and basic formulas

Degree called the expression of the form: where:

Integer

the degree, the indicator of which is a natural number (i.e., a whole and positive).

Rational

the degree, the indicator of which is negative and fractional numbers.

Irrational

the degree, the indicator of which is an infinite decimal fraction or root.

Properties of degrees

Features of degrees.

• Negative number erected into even degree - number positive.
• Negative number erected into odd degree - number negative.
• A positive number to either degree is the number positive.
• Zero to any degree is equal.
• Any number to zero equal.

Now you need a word ...

How do you need an article? Write down in the comments like or not.

Tell me about your experience in using the properties of degrees.

Perhaps you have questions. Or suggestions.

Write in the comments.

And good luck on the exams!

On the channel on YouTube our site site to keep abreast of all new video lessons.

First, let's remember the basic formulas of the degrees and their properties.

The work of the number a. The itself occurs n times, this expression we can write down as a a A ... a \u003d a n

1. A 0 \u003d 1 (A ≠ 0)

3. a n a m \u003d a n + m

4. (a n) m \u003d a nm

5. A N B n \u003d (AB) N

7. A N / A M \u003d A N - M

Power or indicative equations - These are equations in which variables are in degrees (or indicators), and the basis is the number.

Examples of indicative equations:

IN this example Number 6 is the basis it always stands downstairs, but variable x. degree or indicator.

Let us give more examples of the indicative equations.
2 x * 5 \u003d 10
16 x - 4 x - 6 \u003d 0

Now we will analyze how the demonstration equations are solved?

Take a simple equation:

2 x \u003d 2 3

This example can be solved even in the mind. It can be seen that x \u003d 3. After all, so that the left and right part should be equal to the number 3 instead of x.
Now let's see how it is necessary to issue this decision:

2 x \u003d 2 3
x \u003d 3.

In order to solve such an equation, we removed same grounds (i.e. two) and recorded what remains, it is degrees. Received the desired answer.

Now summarize our decision.

Algorithm for solving an indicative equation:
1. Need to check the same Lee foundations at the equation on the right and left. If the bases are not the same as looking for options for solving this example.
2. After the foundations become the same, equal degrees and solve the resulting new equation.

Now rewrite a few examples:

Let's start with a simple.

The bases in the left and right part are equal to Number 2, which means we can reject and equate their degrees.

x + 2 \u003d 4 It turned out the simplest equation.
x \u003d 4 - 2
x \u003d 2.
Answer: x \u003d 2

IN next example It can be seen that the foundations are different this 3 and 9.

3 3x - 9 x + 8 \u003d 0

To begin with, we transfer the nine to the right side, we get:

Now you need to make the same foundation. We know that 9 \u003d 3 2. We use the degree formula (a n) m \u003d a nm.

3 3x \u003d (3 2) x + 8

We obtain 9 x + 8 \u003d (3 2) x + 8 \u003d 3 2x + 16

3 3x \u003d 3 2x + 16 Now it is clear that in the left and right side of the base the same and equal to the troika, which means we can discard them and equate degrees.

3x \u003d 2x + 16 Received the simplest equation
3x - 2x \u003d 16
x \u003d 16.
Answer: X \u003d 16.

We look at the following example:

2 2x + 4 - 10 4 x \u003d 2 4

First, we look at the base, the foundations are different two and four. And we need to be the same. We convert the four by the formula (a n) m \u003d a nm.

4 x \u003d (2 2) x \u003d 2 2x

And also use one formula a n a m \u003d a n + m:

2 2x + 4 \u003d 2 2x 2 4

Add to equation:

2 2x 2 4 - 10 2 2x \u003d 24

We led an example to the same reasons. But we interfere with other numbers 10 and 24. What to do with them? If you can see that it is clear that we have 2 2 2, that's the answer - 2 2, we can take out the brackets:

2 2x (2 4 - 10) \u003d 24

We calculate the expression in brackets:

2 4 — 10 = 16 — 10 = 6

All equation Delim to 6:

Imagine 4 \u003d 2 2:

2 2x \u003d 2 2 bases are the same, throwing out them and equate degrees.
2x \u003d 2 It turned out the simplest equation. We divide it on 2
x \u003d 1.
Answer: x \u003d 1.

Resolving equation:

9 x - 12 * 3 x + 27 \u003d 0

We transform:
9 x \u003d (3 2) x \u003d 3 2x

We get the equation:
3 2x - 12 3 x +27 \u003d 0

The foundations we have the same are equal to three. In this example, it can be seen that the first three degree twice (2x) is greater than that of the second (simply x). In this case, you can solve replacement method. Number C. the smallest degree We replace:

Then 3 2x \u003d (3 x) 2 \u003d T 2

We replace in equation all degrees with cavities on T:

t 2 - 12T + 27 \u003d 0
Receive quadratic equation. We decide through the discriminant, we get:
D \u003d 144-108 \u003d 36
T 1 \u003d 9
T 2 \u003d 3

Return to the variable x..

Take T 1:
T 1 \u003d 9 \u003d 3 x

That is,

3 x \u003d 9
3 x \u003d 3 2
x 1 \u003d 2

One root found. We are looking for the second, from T 2:
T 2 \u003d 3 \u003d 3 x
3 x \u003d 3 1
x 2 \u003d 1
Answer: x 1 \u003d 2; x 2 \u003d 1.

On the site you can in the Help Resolve Decision to ask you ask questions. We will answer.

Join the group

Formulas degrees Used in the process of abbreviation and simplification complex expressions, solving equations and inequalities.

Number c. is an n.Little degree a. when:

Operations with degrees.

1. Multiplying the degree with the same basis, their indicators fold:

a M.· A n \u003d a m + n.

2. In dividing degrees with the same basis, their indicators are deducted:

3. The degree of work 2 or more Multiplers equals the work of the degrees of these factors:

(ABC ...) n \u003d a n · b n · C n ...

4. The degree of fraction is equal to the ratio of degrees of the divide and divider:

(A / B) n \u003d a n / b n.

5. Earring the degree to the degree, the indicators of degrees are prolonged:

(A m) n \u003d a m n.

Each above formula is true in directions from left to right and vice versa.

for example. (2 · 3 · 5/15) ² \u003d 2² · 3² · 5² / 15 ² \u003d 900/225 \u003d 4.

Root operations.

1. The root of the work of several factors is equal to the product of the roots of these factors:

2. The root of the relationship is equal to the attitude of the divide and divider of the roots:

3. When the root is erected, it is fairly built into this degree.

4. If you increase the degree of root in n. once and at the same time build in n.The degree of the feed number, the value of the root will not change:

5. If you reduce the root degree in n. once and at the same time extract the root n.degree from an undercurned number, the value of the root will not change:

Degree with a negative indicator.The degree of a certain number with an indisputable (whole) indicator is determined as a unit divided by the degree of the same number with an indicator equal to the absolute value of the non-positive indicator:

Formula a M.: a n \u003d a m - n can be used not only at m.> n. but also m.< n..

for example. a. 4: A 7 \u003d A 4 \u200b\u200b- 7 \u003d A -3.

To formula a M.: a n \u003d a m - n became fair as m \u003d N.The presence of a zero degree is needed.

The degree with the zero indicator.The degree of any number that is not equal to zero, with the zero indicator equals one.

for example. 2 0 = 1,(-5) 0 = 1,(-3/5) 0 = 1.

Degree with fractional indicator.To build a valid number but in degree m / N., it is necessary to extract the root n.degree from m.degree of this number but.

Type of lesson: Lesson of generalization and systematization of knowledge

Objectives:

• educational - repeat the determination of the degree, the rules of multiplication and division of degrees, the construction of a degree to the degree, consolidate the ability to solve examples containing degrees
• developing - development of logical thinking of students, interest in the material studied,
• raising - Education of a responsible attitude towards studying, culture of communication, feelings of collectivism.

Equipment:computer, multimedia projector, interactive board, Presentation of "degree" for oral account, cards with tasks, distribution material.

Lesson plan:

1. Organizing time.
2. Repetition of rules
3. Verbal counting.
4. Historical reference.
5. Work at the board.
6. Fizkultminutka.
7. Work on an interactive board.
8. Independent work.
9. Homework.
10. Summing up the lesson.

During the classes

I. Organizational moment

Message themes and lesson purposes.

In previous lessons you discovered for yourself amazing world degrees, learned to multiply and share degrees, erect them into a degree. Today we must consolidate the knowledge gained in solving examples.

II. Repetition of rules(orally)

1. Give the definition with a natural indicator? (Degree of numbers but with a natural indicator, large 1, called a work n. multipliers, each of which is equal but.)
2. How to multiply two degrees? (To multiply degrees with identical grounds, It is necessary to leave the base the same, and the indicators are folded.)
3. How to divide the degree to the degree? (To divide degrees with the same bases, it is necessary to leave the base the same, and the indicators subtract.)
4. How to build a product into a degree? (To build a product to a degree, it is necessary every multiplier to this degree)
5. How to build a degree in a degree? (To build a degree to a degree, it is necessary to leave the ground to the same, and multiply indicators)

III. Verbal counting(Multimedia)

IV. Historical reference

All tasks from Papyrus Akhmes, which is recorded about 1650 BC. e. Associated with the practice of construction, the placement of land plots, etc. Tasks are grouped on topics. The advantage is the task of finding the area of \u200b\u200btriangle, four-triggers and a circle, a variety of activities with integers and fractions, proportional division, finding relationships, there is also the construction of various degrees, the solution of equations of the first and second degree with one unknown.

There are no any explanation or evidence. The desired result is either given directly or a brief algorithm for its calculation is given. This method of presentation, typical of science countries of the East, suggests that mathematics there developed by generalizations and guesses that do not form any common theory. Nevertheless, there are in papyrus whole line Evidence that Egyptian mathematicians knew how to extract roots and raise into a degree, solve equations, and even owned the attacks of algebra.

V. Work at the board

Find the value of the expression rational way:

Calculate the value of the expression:

Vi. Fizkultminutka

1. for eyes
2. for neck
3. for hands
4. for Torch
5. for legs

VII. Solving tasks(with a display on an interactive board)

Is the root of the equation of a positive number?

a) 3x + (-0,1) 7 \u003d (-0.496) 4 (x\u003e 0)

b) (10,381) 5 \u003d (-0,012) 3 - 2x (x< 0)

VIII. Independent work

IX. Homework

H. Summing up the lesson

Analysis of the results, declarations.

We will apply the knowledge about degrees in solving equations, tasks in high schools, they are also often found in the exam.

Sections: Mathematics

Type of lesson: Lesson of generalization and systematization of knowledge

Objectives:

educational - repeat the determination of the degree, the rules of multiplication and division of degrees, the construction of a degree to the degree, consolidate the ability to solve examples containing degrees

developing - development of logical thinking of students, interest in the material studied,

raising - Education of a responsible attitude towards studying, culture of communication, feelings of collectivism.

Equipment: Computer, multimedia projector, interactive board, presentation "degree" for oral account, cards with assignments, distribution material.

Lesson plan:

Organizing time.

Repetition of rules

Verbal counting.

Historical reference.

Work at the board.

Fizkultminutka.

Work on an interactive board.

Independent work.

Homework.

Summing up the lesson.

During the classes

I. Organizational moment

Message themes and lesson purposes.

In previous lessons, you discovered the amazing world of degrees, learned to multiply and share degrees, erect them into a degree. Today we must consolidate the knowledge gained in solving examples.

II. Repetition of rules (orally)

1. Give the definition with a natural indicator? (Degree of numbers but with a natural indicator, large 1, called a work n. multipliers, each of which is equal but.)
2. How to multiply two degrees? (To multiply degrees with the same bases, it is necessary to leave the base in the same way, and the indicators are folded.)
3. How to divide the degree to the degree? (To divide degrees with the same bases, it is necessary to leave the base the same, and the indicators subtract.)
4. How to build a product into a degree? (To build a product to a degree, it is necessary every multiplier to this degree)
5. How to build a degree in a degree? (To build a degree to a degree, it is necessary to leave the ground to the same, and multiply indicators)

III. Verbal counting (Multimedia)

IV. Historical reference

All tasks from Papyrus Akhmes, which is recorded about 1650 BC. e. Associated with the practice of construction, the placement of land plots, etc. Tasks are grouped on topics. The advantage is the task of finding the area of \u200b\u200btriangle, four-triggers and a circle, a variety of activities with integers and fractions, proportional division, finding relationships, there is also the construction of various degrees, the solution of equations of the first and second degree with one unknown.

There are no any explanation or evidence. The desired result is either given directly or a brief algorithm for its calculation is given. This method of presentation, typical of the science of the countries of the Ancient East, suggests that mathematics develops there by generalizations and guesses that do not form any common theory. However, in papyrus there are a number of evidence that Egyptian mathematicians knew how to extract roots and raise the degree, solve equations, and even owned the attacks of algebra.

V. Work at the board

Find the value of the expression rational way:

Calculate the value of the expression:



Vi. Fizkultminutka

6. for eyes
7. for neck
8. for hands
9. for Torch
10. for legs

VII. Solving tasks (with a display on an interactive board)

Is the root of the equation of a positive number?

xN - i1abbnckbmcl9fb.xn - P1AI

The formulas of degrees and roots.

Formulas degrees Used in the process of abbreviation and simplify complex expressions, in solving equations and inequalities.

Number c. is an n.Little degree a. when:



Operations with degrees.

1. Multiplying the degree with the same basis, their indicators fold:

2. In dividing degrees with the same basis, their indicators are deducted:



3. The degree of work of 2 or more multipliers is equal to the product of these factors:

(ABC ...) n \u003d a n · b n · C n ...

4. The degree of fraction is equal to the ratio of degrees of the divide and divider:

5. Earring the degree to the degree, the indicators of degrees are prolonged:

Each above formula is true in directions from left to right and vice versa.

Root operations.

1. The root of the work of several factors is equal to the product of the roots of these factors:

2. The root of the relationship is equal to the attitude of the divide and divider of the roots:



3. When the root is erected, it is fairly built into this degree.



4. If you increase the degree of root in n. once and at the same time build in n.The degree of the feed number, the value of the root will not change:



5. If you reduce the root degree in n. once and at the same time extract the root n.degree from an undercurned number, the value of the root will not change:



The degree of a certain number with an indisputable (whole) indicator is determined as a unit divided by the degree of the same number with an indicator equal to the absolute value of the non-positive indicator:



Formula a M. : a n \u003d a m - n can be used not only at m. > n. but also m. 4: A 7 \u003d A 4 \u200b\u200b- 7 \u003d A -3.

To formula a M. : a n \u003d a m - n became fair as m \u003d N.The presence of a zero degree is needed.

The degree of any number that is not equal to zero, with the zero indicator equals one.

To build a valid number but in degree m / N., it is necessary to extract the root n.Degree from m.degree of this number but:



Formulas degrees.

6. a. — n. = - division of degrees;

7. - division of degrees;

8. A 1 / N \u003d ;

Degree rules of action with degrees

1. The degree of work of two or several womb is equal to the work of the degrees of these factors (with the same indicator):

(ABC ...) n \u003d a n b n c n ...

Example 1. (7 2 10) 2 \u003d 7 2 2 2 10 2 \u003d 49 4 100 \u003d 19600. Example 2. (x 2 -a 2) 3 \u003d [(x + a) (x - a)] 3 \u003d ( x + a) 3 (x - a) 3

Almost important reverse transformation:

a n b n c n ... \u003d (abc ...) n

those. The product of the same degrees of several quantities is equal to the same degree of the product of these values.

Example 3. Example 4. (A + B) 2 (A 2 - AB + B 2) 2 \u003d [(A + B) (A 2 - AB + B 2)] 2 \u003d (A 3 + B 3) 2

2. The degree of private (fracted) is equal to the private from dividing the same degree divided by the same degree of the divider:

Example 5. Example 6.

Reverse transformation:. Example 7. . Example 8. .

3. When multiplying degrees with the same bases, degrees are folded:

Example 9.2 2 2 5 \u003d 2 2 + 5 \u003d 2 7 \u003d 128. Example 10. (A - 4C + X) 2 (A - 4C + X) 3 \u003d (A - 4C + X) 5.

4. When dividing degrees with the same bases, the degree of divider is deducted from the degree of divide

Example 11. 12 5:12 3 \u003d 12 5-3 \u003d 12 2 \u003d 144. Example 12. (X-y) 3: (x - y) 2 \u003d x-y.

5. When erecting the degree to the degree, the degree indicators are variable:

Example 13. (2 3) 2 \u003d 2 6 \u003d 64. Example 14.

www.maths.yfa1.ru.

Degrees and roots

Operations with degrees and roots. Degree with negative ,

zero and fractional indicator. About expressions that do not make sense.

Operations with degrees.

1. When multiplying degrees with the same base, their indicators fold:

a M. · a n \u003d a m + n.

2. When dividing degrees with the same basis, their indicators remove .



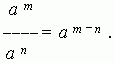
3. The degree of work of two or several woggles is equal to the work of the degrees of these factors.

4. The degree of relation (fracted) is equal to the ratio of degrees of the divide (numerator) and the divider (denominator):

(a / B.) n \u003d a n / b n.

5. When erecting a degree to the degree, their indicators are multiplied:

All of the above formulas are read and are performed in both directions from left to right and vice versa.

PRI MERS (2 · 3 · 5/15) ² = 2 ² · 3 ² · 5 ² / 15 ² \u003d 900/225 \u003d 4 .

Root operations. In all the following formulas, the symbol means arithmetic root (Courted expression positively).

1. The root of the work of several womb is equal to the product of the roots of these factors:

2. The root from the relationship is equal to the attitude of the roots of the divide and divider:



3. When the root is erected, it is enough to build this degree subject:



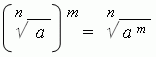
4. If you increase the degree of root in M \u200b\u200btimes and at the same time build a feed number into a m-degree, the root value will not change:



5. If you reduce the degree of root in M \u200b\u200btimes and at the same time remove the root of the M-degree from the feed number, the root value will not change:




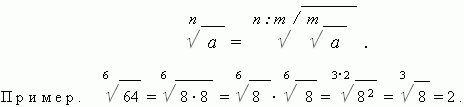
Expansion of the concept of degree. So far, we have considered degrees only with a natural indicator; But the actions with degrees and roots can also lead to negative, zero and fractional Indicators. All these indicators of degrees require additional definition.

Degree with a negative indicator. The degree of a certain number with a negative (whole) indicator is defined as a unit divided by the degree of the same number with an indicator equal to the absolute veliver of the negative indicator:



T Heathe formula a M. : a N. = a M - N can be used not only at m. more than n. but also m. less than n. .

PRI MERS a. 4: a. 7 \u003d A. 4 — 7 \u003d A. — 3 .

If we want the formula a M. : a N. = a M. — n. It was fair for m \u003d N. We need to determine zero degree.

The degree with the zero indicator. The degree of any nonzero number with zero is equal to 1.

PRI MERS. 2 0 \u003d 1, ( – 5) 0 = 1, (– 3 / 5) 0 = 1.

Degree with fractional indicator. In order to build a valid number A into the degree M / N, it is necessary to extract the root of the N-degree from M-degree of this number A:



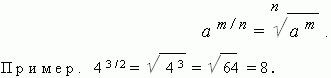
About expressions that do not make sense. There are several such expressions.

where a. ≠ 0 , does not exist.

In fact, assuming that x. - Some number, then in accordance with the definition of the division operation, we have: a. = 0· x.. a. \u003d 0, which contradicts the condition: a. ≠ 0

— any number.

In fact, assuming that this expression is equal to some number x., according to the definition of the division operation, we have: 0 \u003d 0 · x. . But this equality takes place when any number X.As required to prove.

0 0 — any number.



Consider three fundamental cases:

1) x. = 0 – This value does not satisfy this equation.

2) for x. \u003e 0 We get: x / X. \u003d 1, i.e. 1 \u003d 1, from where it follows

what x. - any number; But taking into account that in

oUR Case x. \u003e 0, answer is x. > 0 ;

Properties of degree

We remind you that in this lesson you understand properties of degrees with natural indicators and zero. The degrees with rational indicators and their properties will be considered in lessons for 8 classes.

The ratio with a natural indicator has several important properties that allow you to simplify calculations in examples with degrees.

Property number 1.
The work of degrees

When multiplying degrees with the same bases, the base remains unchanged, and the indicators of degrees are folded.

a m · a n \u003d a m + n, where "A" is any number, and "M", "N" - any natural numbers.

This property of degrees also acts on the work of three and more degrees.

• Simplify the expression.
  b · b 2 · b 3 · b 4 · b 5 \u003d b 1 + 2 + 3 + 4 + 5 \u003d b 15
• Represent in the form of degree.
  6 15 · 36 \u003d 6 15 · 6 2 \u003d 6 15 · 6 2 \u003d 6 17
• Represent in the form of degree.
  (0.8) 3 · (0.8) 12 \u003d (0.8) 3 + 12 \u003d (0.8) 15

Please note that in specified property It was only about multiplying degrees with the same foundations. . It does not apply to their addition.

It is impossible to replace the amount (3 3 + 3 2) by 3 5. This is understandable if
calculate (3 3 + 3 2) \u003d (27 + 9) \u003d 36, a 3 5 \u003d 243

Property number 2.
Private degree

When dividing degrees with the same bases, the base remains unchanged, and from the indicator of the division deductible the degree of divider.

• Write private in the form of degree
  (2b) 5: (2b) 3 \u003d (2b) 5 - 3 \u003d (2b) 2
• Calculate.

11 3 - 2 · 4 2 - 1 \u003d 11 · 4 \u003d 44
Example. Solve equation. We use the property of private degrees.
3 8: T \u003d 3 4

Answer: T \u003d 3 4 \u003d 81

Using properties No. 1 and No. 2, you can easily simplify expressions and make calculations.

Example. Simplify the expression.
4 5m + 6 · 4 m + 2: 4 4m + 3 \u003d 4 5m + 6 + m + 2: 4 4m + 3 \u003d 4 6m + 8 - 4m - 3 \u003d 4 2m + 5

Example. Find the value of the expression using the degree properties.

2 11 − 5 = 2 6 = 64

Please note that in the property 2 it was only about dividing degrees with the same bases.

It is impossible to replace the difference (4 3 -4 2) by 4 1. This is understandable if you calculate (4 3 -4 2) \u003d (64 - 16) \u003d 48, a 4 1 \u003d 4

Property number 3.
Erect

When erecting the degree to the degree, the foundation remains unchanged, and the indicators of degrees are variable.

(a n) m \u003d a n · m, where "A" is any number, and "M", "N" - any natural numbers.

Example.
(A 4) 6 \u003d A 4 \u200b\u200b· 6 \u003d A 24

Example. Present 3 20 in the form of a degree with a base of 3 2.

By the field of exercise to the degree It is known that when the degree is raised, the indicators are variable, it means:

Properties 4.
Degree of work

When erecting the degree into the degree of work, each multiplier is erected into this degree, and the results are multiplied.

(a · b) n \u003d a n · b n, where "a", "b" - any rational numbers; "N" - any natural number.

• Example 1.
  (6 · a 2 · b 3 · c) 2 \u003d 6 2 · a 2 · 2 · b 3 · 2 · C 1 · 2 \u003d 36 A 4 · B 6 · C 2
• Example 2.
  (-X 2 · y) 6 \u003d ((-1) 6 · x 2 · 6 · y 1 · 6) \u003d x 12 · y 6

Please note that property number 4, as well as other properties of degrees, apply in reverse order.

(a n · b n) \u003d (a · b) n

That is, in order to multiply the degrees with the same indicators, it is possible to multiply the bases, and the degree indicator is unchanged.

• Example. Calculate.
  2 4 · 5 4 \u003d (2 · 5) 4 \u003d 10 4 \u003d 10 000
• Example. Calculate.
  0.5 16 · 2 16 \u003d (0,5 · 2) 16 \u003d 1

In more complex examples There may be cases when multiplication and division must be performed above degrees with different bases and different indicators. In this case, we recommend to act as follows.

For example, 4 5 · 3 2 \u003d 4 3 · 4 2 · 3 2 \u003d 4 3 · (4 · 3) 2 \u003d 64 · 12 2 \u003d 64 · 144 \u003d 9216

Example of decimal fraction.

4 21 · (-0.25) 20 \u003d 4 · 4 20 · (-0.25) 20 \u003d 4 · (4 · (-0.25)) 20 \u003d 4 · (-1) 20 \u003d 4 · 1 \u003d four

Properties 5.
Private degree (fraction)

To invite the degree in private, you can build a separate and divider into this degree, and the first result is divided into the second.

(A: b) n \u003d a n: b n, where "a", "b" - any rational numbers, b ≠ 0, n - any natural number.

• Example. Present an expression in the form of private degrees.
  (5: 3) 12 = 5 12: 3 12

We remind you that private can be represented as a fraction. Therefore, on the topic, we will focus more in more detail on the next page.