Derivative 2x root of x. Derivative of a complex function. Examples of solutions
Definition. Let the function \ (y = f (x) \) be defined in some interval containing the point \ (x_0 \). Give the argument an increment \ (\ Delta x \) such that it does not go out of this interval. Find the corresponding function increment \ (\ Delta y \) (when passing from point \ (x_0 \) to point \ (x_0 + \ Delta x \)) and compose the ratio \ (\ frac (\ Delta y) (\ Delta x) \). If there is a limit of this ratio at \ (\ Delta x \ rightarrow 0 \), then the specified limit is called derivative function\ (y = f (x) \) at the point \ (x_0 \) and denote \ (f "(x_0) \).
$$ \ lim _ (\ Delta x \ to 0) \ frac (\ Delta y) (\ Delta x) = f "(x_0) $$
The symbol y "is often used to denote the derivative. Note that y" = f (x) is a new function, but naturally related to the function y = f (x), defined at all points x at which the above limit exists ... This function is called like this: derivative of the function y = f (x).
The geometric meaning of the derivative is as follows. If the graph of the function y = f (x) at a point with abscissa x = a can be drawn tangent, not parallel to the y-axis, then f (a) expresses the slope of the tangent:
\ (k = f "(a) \)
Since \ (k = tg (a) \), the equality \ (f "(a) = tg (a) \) is true.
Now let us interpret the definition of the derivative from the point of view of approximate equalities. Let the function \ (y = f (x) \) have a derivative at a specific point \ (x \):
$$ \ lim _ (\ Delta x \ to 0) \ frac (\ Delta y) (\ Delta x) = f "(x) $$
This means that the approximate equality \ (\ frac (\ Delta y) (\ Delta x) \ approx f "(x) \) is fulfilled near the point x, i.e. \ (\ Delta y \ approx f" (x) \ cdot \ Delta x \). The meaningful meaning of the obtained approximate equality is as follows: the increment of the function is "almost proportional" to the increment of the argument, and the coefficient of proportionality is the value of the derivative at a given point x. For example, the function \ (y = x ^ 2 \) satisfies the approximate equality \ (\ Delta y \ approx 2x \ cdot \ Delta x \). If we carefully analyze the definition of the derivative, we will find that it contains an algorithm for finding it.
Let's formulate it.
How to find the derivative of the function y = f (x)?
1. Fix the value \ (x \), find \ (f (x) \)
2. Give the argument \ (x \) an increment \ (\ Delta x \), go to a new point \ (x + \ Delta x \), find \ (f (x + \ Delta x) \)
3. Find the increment of the function: \ (\ Delta y = f (x + \ Delta x) - f (x) \)
4. Make up the relation \ (\ frac (\ Delta y) (\ Delta x) \)
5. Calculate $$ \ lim _ (\ Delta x \ to 0) \ frac (\ Delta y) (\ Delta x) $$
This limit is the derivative of the function at the point x.
If the function y = f (x) has a derivative at the point x, then it is called differentiable at the point x. The procedure for finding the derivative of a function y = f (x) is called differentiation function y = f (x).
Let us discuss the following question: how are the continuity and differentiability of a function at a point related to each other?
Let the function y = f (x) be differentiable at the point x. Then a tangent can be drawn to the graph of the function at the point M (x; f (x)), and, recall, the slope of the tangent is equal to f "(x). Such a graph cannot" break "at the point M, that is, the function must be continuous at point x.
It was a "fingertip" reasoning. Let us give a more rigorous reasoning. If the function y = f (x) is differentiable at the point x, then the approximate equality \ (\ Delta y \ approx f "(x) \ cdot \ Delta x \) holds. If in this equality \ (\ Delta x \) tends to zero, then \ (\ Delta y \) will tend to zero, and this is the condition for the continuity of the function at the point.
So, if the function is differentiable at the point x, then it is also continuous at this point.
The converse is not true. For example: function y = | x | is continuous everywhere, in particular at the point x = 0, but the tangent to the graph of the function at the "junction point" (0; 0) does not exist. If at some point the tangent cannot be drawn to the graph of the function, then there is no derivative at this point.
One more example. The function \ (y = \ sqrt (x) \) is continuous on the whole number line, including at the point x = 0. And the tangent to the graph of the function exists at any point, including at the point x = 0. But at this point the tangent coincides with the y-axis, that is, it is perpendicular to the abscissa axis, its equation has the form x = 0. There is no slope for such a straight line, so there is no \ (f "(0) \)
So, we got acquainted with a new property of a function - differentiability. And how, from the graph of the function, can we conclude about its differentiability?
The answer is actually received above. If at some point to the graph of the function it is possible to draw a tangent that is not perpendicular to the abscissa axis, then at this point the function is differentiable. If at some point the tangent to the graph of the function does not exist or it is perpendicular to the abscissa axis, then at this point the function is not differentiable.
Differentiation rules
The operation of finding the derivative is called differentiation... When performing this operation, you often have to work with quotients, sums, products of functions, as well as with "function functions", that is, complex functions. Based on the definition of a derivative, it is possible to derive differentiation rules that facilitate this work. If C is a constant number and f = f (x), g = g (x) are some differentiable functions, then the following are true differentiation rules:
$$ f "_x (g (x)) = f" _g \ cdot g "_x $$
Derivative table of some functions
$$ \ left (\ frac (1) (x) \ right) "= - \ frac (1) (x ^ 2) $$ $$ (\ sqrt (x))" = \ frac (1) (2 \ sqrt (x)) $$ $$ \ left (x ^ a \ right) "= ax ^ (a-1) $$ $$ \ left (a ^ x \ right)" = a ^ x \ cdot \ ln a $$ $$ \ left (e ^ x \ right) "= e ^ x $$ $$ (\ ln x)" = \ frac (1) (x) $$ $$ (\ log_a x) "= \ frac (1) (x \ ln a) $$ $$ (\ sin x) "= \ cos x $$ $$ (\ cos x)" = - \ sin x $$ $$ (\ text (tg) x) "= \ frac (1) (\ cos ^ 2 x) $$ $$ (\ text (ctg) x)" = - \ frac (1) (\ sin ^ 2 x) $$ (\ arcsin x) "= \ frac (1) (\ sqrt (1-x ^ 2)) $$ $$ (\ arccos x)" = \ frac (-1) (\ sqrt (1-x ^ 2)) $$ $$ (\ text (arctg) x) "= \ frac (1) (1 + x ^ 2) $$ $$ (\ text (arcctg) x)" = \ frac (-1) (1 + x ^ 2) $ $It is absolutely impossible to solve physical problems or examples in mathematics without knowledge of the derivative and the methods of calculating it. Derivative is one of the most important concepts of mathematical analysis. We decided to devote today's article to this fundamental topic. What is a derivative, what is its physical and geometric meaning, how to calculate the derivative of a function? All these questions can be combined into one: how to understand the derivative?
Geometric and physical meaning of the derivative
Let there be a function f (x) given in some interval (a, b) ... Points х and х0 belong to this interval. When x changes, the function itself changes. Changing an argument - the difference between its values x-x0 ... This difference is written as delta x and is called argument increment. A change or increment of a function is the difference in the values of a function at two points. Derivative definition:
The derivative of a function at a point is the limit of the ratio of the increment of the function at a given point to the increment of the argument when the latter tends to zero.
Otherwise, it can be written like this:
What's the point in finding such a limit? And here's what:
the derivative of the function at a point is equal to the tangent of the angle between the OX axis and the tangent to the graph of the function at this point.
The physical meaning of the derivative: the derivative of the path with respect to time is equal to the speed of the rectilinear motion.
Indeed, since school times, everyone knows that speed is a private path. x = f (t) and time t ... Average speed over a period of time:
To find out the speed of movement at a time t0 you need to calculate the limit:
Rule one: take out a constant
The constant can be moved outside the sign of the derivative. Moreover, it must be done. When solving examples in math, take as a rule - if you can simplify the expression, be sure to simplify .
Example. Let's calculate the derivative:
Rule two: derivative of the sum of functions
The derivative of the sum of two functions is equal to the sum of the derivatives of these functions. The same is true for the derivative of the difference of functions.
We will not give a proof of this theorem, but rather consider a practical example.
Find the derivative of a function:
Rule three: derivative of the product of functions
The derivative of the product of two differentiable functions is calculated by the formula:
Example: find the derivative of a function:
Solution: 
It is important to say here about the calculation of derivatives of complex functions. The derivative of a complex function is equal to the product of the derivative of this function with respect to the intermediate argument by the derivative of the intermediate argument with respect to the independent variable.
In the above example, we meet the expression:
In this case, the intermediate argument is 8x to the fifth power. In order to calculate the derivative of such an expression, we first calculate the derivative of the external function with respect to the intermediate argument, and then multiply by the derivative of the immediate intermediate argument with respect to the independent variable.
Rule four: the quotient derivative of two functions
Formula for determining the derivative of the quotient of two functions:
We tried to tell you about derivatives for dummies from scratch. This topic is not as simple as it sounds, so be warned: there are often pitfalls in the examples, so be careful when calculating derivatives.
For any question on this and other topics, you can contact the student service. Per short term we can help you solve the most difficult test and solve tasks, even if you have never done calculating derivatives before.
On which we analyzed the simplest derivatives, and also got acquainted with the rules of differentiation and some techniques for finding derivatives. Thus, if you are not very good with derivatives of functions, or some points of this article are not entirely clear, then first read the above lesson. Please, tune in to a serious mood - the material is not an easy one, but I will try to present it simply and easily.
In practice, you have to deal with the derivative of a complex function very often, I would even say, almost always, when you are given tasks to find derivatives.
We look in the table at the rule (No. 5) for differentiating a complex function:
Understanding. First of all, let's pay attention to the recording. Here we have two functions - and, moreover, the function, figuratively speaking, is embedded in the function. A function of this kind (when one function is nested within another) is called a complex function.
I will call the function external function and the function - an inner (or nested) function.
! These definitions are not theoretical and should not appear in the final design of the assignments. I use informal expressions "external function", "internal" function only to make it easier for you to understand the material.
In order to clarify the situation, consider:
Example 1
Find the derivative of a function
Under the sine we have not just the letter "X", but an integer expression, so it will not be possible to find the derivative immediately from the table. We also notice that it is impossible to apply the first four rules here, there seems to be a difference, but the fact is that it is impossible to "tear apart" a sine:
In this example, already from my explanations, it is intuitively clear that a function is a complex function, and the polynomial is an internal function (nesting), and an external function.
First step, which must be performed when finding the derivative of a complex function, is that figure out which function is internal and which is external.
When simple examples it seems clear that a polynomial is nested under the sine. But what if everything is not obvious? How to determine exactly which function is external and which is internal? To do this, I suggest using the following technique, which can be done mentally or on a draft.
Imagine that we need to calculate the value of an expression at on a calculator (instead of one, there can be any number).
What will we calculate first? First of all you will need to perform the following action:, so the polynomial will be an internal function: 
Secondary will need to be found, so sine will be an external function: 
After we Figured out with internal and external functions, it's time to apply the rule of differentiation of a complex function 
.
We start to decide. From the lesson How do I find the derivative? we remember that the design of the solution of any derivative always begins like this - we enclose the expression in parentheses and put a stroke on the top right:
At first find the derivative of the external function (sine), look at the table of derivatives of elementary functions and notice that. All tabular formulas are applicable even if "x" is replaced with a complex expression, in this case:
Note that the inner function has not changed, we do not touch it.
Well, it is quite obvious that
The result of applying the formula 
in the final design it looks like this:
The constant factor is usually placed at the beginning of the expression:
If there is any confusion, write the solution down and read the explanations again.
Example 2
Find the derivative of a function
Example 3
Find the derivative of a function
As always, we write down: 
Let's figure out where we have an external function, and where we have an internal one. To do this, try (mentally or on a draft) to calculate the value of the expression at. What should be done first? First of all, you need to calculate what the base is equal to: which means that the polynomial is the internal function: 
And, only then the exponentiation is performed, therefore, power function Is an external function: 
According to the formula 
, first you need to find the derivative of the external function, in this case, from the degree. We are looking for the required formula in the table:. We repeat again: any tabular formula is valid not only for "x", but also for a complex expression... Thus, the result of applying the rule of differentiation of a complex function 
next:
I emphasize again that when we take the derivative of the outer function, the inner function does not change for us: 
Now it remains to find a very simple derivative of the inner function and "comb" the result a little:
Example 4
Find the derivative of a function
This is an example for independent decision(answer at the end of the lesson).
To consolidate the understanding of the derivative of a complex function, I will give an example without comments, try to figure it out on your own, speculate where is the external and where is the internal function, why the tasks were solved that way?
Example 5
a) Find the derivative of the function
b) Find the derivative of the function
Example 6
Find the derivative of a function 
Here we have a root, and in order to differentiate the root, it must be represented as a degree. Thus, first we bring the function into a form appropriate for differentiation:
Analyzing the function, we come to the conclusion that the sum of three terms is an internal function, and exponentiation is an external function. We apply the rule of differentiation of a complex function 
:
The degree is again represented as a radical (root), and for the derivative of the internal function we apply a simple rule for differentiating the sum:
Ready. You can also bring the expression to a common denominator in parentheses and write everything down in one fraction. Nice, of course, but when cumbersome long derivatives are obtained, it is better not to do this (it is easy to get confused, make an unnecessary mistake, and it will be inconvenient for the teacher to check).
Example 7
Find the derivative of a function
This is an example for a do-it-yourself solution (answer at the end of the tutorial).
It is interesting to note that sometimes, instead of the rule for differentiating a complex function, one can use the rule for differentiating the quotient 
, but such a solution will look unusual as a perversion. Here's a typical example:
Example 8
Find the derivative of a function
Here you can use the rule of differentiation of the quotient 
, but it is much more profitable to find the derivative through the rule of differentiation of a complex function:
We prepare the function for differentiation - we move the minus behind the sign of the derivative, and raise the cosine to the numerator:
Cosine is an internal function, exponentiation is an external function.
We use our rule 
:
Find the derivative of the internal function, reset the cosine back down:
Ready. In the considered example, it is important not to get confused in the signs. By the way, try to solve it with the rule 
, the answers must match.
Example 9
Find the derivative of a function
This is an example for a do-it-yourself solution (answer at the end of the tutorial).
So far, we've looked at cases where we only had one attachment in a complex function. In practical tasks, you can often find derivatives, where, like nesting dolls, one into another, 3 or even 4-5 functions are nested at once.
Example 10
Find the derivative of a function
Let's understand the attachments of this function. Trying to evaluate the expression using the test value. How would we count on a calculator?
First you need to find, which means that the arcsine is the deepest nesting:
Then this arcsine of one should be squared:
And finally, raise the 7 to the power: 
That is, in this example, we have three different functions and two attachments, while the innermost function is the arcsine, and the outermost function is the exponential function.
We start to solve
According to the rule 
first you need to take the derivative of the external function. We look at the table of derivatives and find the derivative exponential function: The only difference is that instead of "X" we have complex expression, which does not negate the validity of this formula. So, the result of applying the rule of differentiation of a complex function 
next.
The operation of finding a derivative is called differentiation.
As a result of solving the problems of finding derivatives of the simplest (and not very simple) functions by determining the derivative as the limit of the ratio of the increment to the increment of the argument, a table of derivatives appeared and exactly certain rules differentiation. The first in the field of finding derivatives were Isaac Newton (1643-1727) and Gottfried Wilhelm Leibniz (1646-1716).
Therefore, in our time, in order to find the derivative of any function, it is not necessary to calculate the above-mentioned limit of the ratio of the increment of the function to the increment of the argument, but you just need to use the table of derivatives and the rules of differentiation. The following algorithm is suitable for finding the derivative.
To find the derivative, you need an expression under the stroke sign disassemble simple functions and determine what actions (product, sum, quotient) these functions are linked. Further, the derivatives of elementary functions are found in the table of derivatives, and the formulas for derivatives of the product, sum and quotient are found in the rules of differentiation. Derivative table and differentiation rules are given after the first two examples.
Example 1. Find the derivative of a function
Solution. From the rules of differentiation, we find out that the derivative of the sum of functions is the sum of the derivatives of functions, i.e.
From the table of derivatives we find out that the derivative of the "x" is equal to one, and the derivative of the sine is equal to the cosine. We substitute these values into the sum of derivatives and find the derivative required by the condition of the problem:
Example 2. Find the derivative of a function
Solution. We differentiate as the derivative of the sum, in which the second term with a constant factor, it can be taken out of the sign of the derivative:
If there are still questions about where what comes from, they, as a rule, become clearer after familiarization with the table of derivatives and the simplest rules of differentiation. We are going to them right now.
Derivative table of simple functions
| 1. Derivative of a constant (number). Any number (1, 2, 5, 200 ...) that is in the function expression. Always zero. This is very important to remember, as it is required very often. | |
| 2. Derivative of the independent variable. Most often "x". Always equal to one. This is also important to remember for a long time. | |
| 3. Derivative degree. When solving problems, you need to transform non-square roots into a power. | |
| 4. Derivative of a variable to the power of -1 | |
| 5. Derivative square root | |
| 6. Derivative of sine | |
| 7. Derivative of the cosine |  |
| 8. Derivative of the tangent |  |
| 9. Derivative of the cotangent |  |
| 10. Derivative of the arcsine |  |
| 11. Derivative of the arccosine |  |
| 12. Derivative of the arctangent |  |
| 13. Derivative of arc cotangent |  |
| 14. Derivative of the natural logarithm | |
| 15. Derivative of the logarithmic function |  |
| 16. Derivative of the exponent | |
| 17. Derivative of the exponential function |
Differentiation rules
| 1. Derivative of the sum or difference |  |
| 2. Derivative of the work |  |
| 2a. Derivative of an expression multiplied by a constant factor | |
| 3. Derivative of the quotient |  |
| 4. Derivative of a complex function |  |
Rule 1.If functions
differentiable at some point, then at the same point the functions
moreover
those. the derivative of the algebraic sum of functions is equal to the algebraic sum of the derivatives of these functions.
Consequence. If two differentiable functions differ by a constant term, then their derivatives are equal, i.e.
Rule 2.If functions
differentiable at some point, then at the same point their product is also differentiable
moreover
those. the derivative of the product of two functions is equal to the sum of the products of each of these functions by the derivative of the other.
Corollary 1. The constant factor can be moved outside the sign of the derivative:
Corollary 2. The derivative of the product of several differentiable functions is equal to the sum of the products of the derivative of each of the factors by all the others.
For example, for three factors:
Rule 3.If functions
differentiable at some point and , then at this point it is differentiable and their quotientu / v, and
those. the derivative of the quotient of two functions is equal to the fraction, the numerator of which is the difference between the products of the denominator and the derivative of the numerator and the numerator by the derivative of the denominator, and the denominator is the square of the previous numerator.
Where what to look for on other pages
When finding the derivative of the product and the quotient in real problems, it is always necessary to apply several differentiation rules at once, therefore, more examples on these derivatives are in the article"Derivative of a work and a particular function".
Comment. Do not confuse a constant (that is, a number) as a summand and as a constant factor! In the case of a term, its derivative is equal to zero, and in the case of a constant factor, it is taken out of the sign of the derivatives. it typical mistake which occurs on initial stage studying derivatives, but as several one- or two-component examples are solved, the average student no longer makes this mistake.
And if, when differentiating a work or a particular, you have a term u"v, in which u- a number, for example, 2 or 5, that is, a constant, then the derivative of this number will be equal to zero and, therefore, the entire term will be equal to zero (this case is analyzed in Example 10).
Other common mistake- mechanical solution of the derivative of a complex function as a derivative of a simple function. That's why derivative of a complex function a separate article is devoted. But first, we will learn to find the derivatives simple functions.
Along the way, you can not do without expression transformations. To do this, you may need to open the tutorials in new windows Actions with powers and roots and Fraction actions .
If you are looking for solutions to derivatives of fractions with powers and roots, that is, when the function looks like 
, then follow the lesson Derivative of the Sum of Fractions with Powers and Roots.
If you have a task like 
, then your lesson "Derivatives of simple trigonometric functions".
Step by step examples - how to find the derivative
Example 3. Find the derivative of a function
Solution. We determine the parts of the function expression: the whole expression represents the product, and its factors are sums, in the second of which one of the terms contains a constant factor. We apply the rule of product differentiation: the derivative of the product of two functions is equal to the sum of the products of each of these functions by the derivative of the other:
Next, we apply the rule for differentiating the sum: the derivative of the algebraic sum of functions is equal to the algebraic sum of the derivatives of these functions. In our case, in each sum, the second term with a minus sign. In each sum we see both an independent variable, the derivative of which is equal to one, and a constant (number), the derivative of which is equal to zero. So, "x" for us turns into one, and minus 5 - into zero. In the second expression, "x" is multiplied by 2, so that two is multiplied by the same unit as the derivative of "x". We get following values derivatives:
We substitute the found derivatives into the sum of products and obtain the derivative of the entire function required by the condition of the problem:
Example 4. Find the derivative of a function
Solution. We are required to find the derivative of the quotient. We apply the formula for differentiating the quotient: the derivative of the quotient of two functions is equal to a fraction, the numerator of which is the difference between the products of the denominator and the derivative of the numerator and the numerator and the derivative of the denominator, and the denominator is the square of the previous numerator. We get:
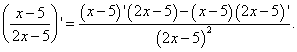
We have already found the derivative of the factors in the numerator in Example 2. Do not forget that the product that is the second factor in the numerator in the current example is taken with a minus sign:
If you are looking for solutions to problems in which you need to find the derivative of a function, where there is a continuous jumble of roots and powers, such as, for example, 
then welcome to class "Derivative of the sum of fractions with powers and roots" .
If you need to learn more about the derivatives of sines, cosines, tangents and others trigonometric functions, that is, when the function looks like , then your lesson "Derivatives of simple trigonometric functions" .
Example 5. Find the derivative of a function
Solution. In this function, we see a product, one of the factors of which is the square root of the independent variable, the derivative of which we familiarized ourselves with in the table of derivatives. According to the rule of differentiation of the product and the tabular value of the derivative of the square root, we obtain:
Example 6. Find the derivative of a function
Solution. In this function, we see the quotient, the dividend of which is the square root of the independent variable. According to the rule of differentiation of the quotient, which we repeated and applied in example 4, and the table value of the derivative of the square root, we get:
To get rid of the fraction in the numerator, multiply the numerator and denominator by.
In this lesson, we will learn how to apply differentiation formulas and rules.
Examples. Find derivatives of functions.
1. y = x 7 + x 5 -x 4 + x 3 -x 2 + x-9. Apply the rule I, formulas 4, 2 and 1... We get:
y '= 7x 6 + 5x 4 -4x 3 + 3x 2 -2x + 1.
2. y = 3x 6 -2x + 5. We solve in a similar way, using the same formulas and the formula 3.
y ’= 3 ∙ 6x 5 -2 = 18x 5 -2.
Apply the rule I, formulas 3, 5
and 6
and 1.
Apply the rule IV, formulas 5 and 1 .
In the fifth example, according to the rule I the derivative of the sum is equal to the sum of the derivatives, and we have just found the derivative of the 1st term (example 4 ), therefore, we will find the derivatives 2nd and 3rd terms, and for the 1st term, we can immediately write the result.
Differentiating 2nd and 3rd terms according to the formula 4
... To do this, we transform the roots of the third and fourth degrees in denominators to degrees with negative exponents, and then, by 4
formula, we find the derivatives of the powers.
Look at given example and the result obtained. Got a pattern? Good. This means that we have a new formula and can add it to our table of derivatives.
Let's solve the sixth example and derive another formula.
We use the rule IV and the formula 4
... Reduce the resulting fractions.
We look at this function and its derivative. You, of course, understood the pattern and are ready to name the formula:
Learning new formulas!
Examples.
1. Find the argument increment and function increment y = x 2 if the initial value of the argument was 4 and new - 4,01 .
Solution.
New argument value x = x 0 + Δx... Substitute the data: 4.01 = 4 + Δx, hence the argument increment Δx= 4.01-4 = 0.01. The increment of a function, by definition, is equal to the difference between the new and previous values of the function, i.e. Δy = f (x 0 + Δx) - f (x 0). Since we have a function y = x 2, then Δy= (x 0 + Δx) 2 - (x 0) 2 = (x 0) 2 + 2x 0 · Δx + (Δx) 2 - (x 0) 2 = 2x 0 · Δx + (Δx) 2 =
2 · 4 · 0,01+(0,01) 2 =0,08+0,0001=0,0801.
Answer: argument increment Δx= 0.01; function increment Δy=0,0801.
It was possible to find the increment of the function in a different way: Δy= y (x 0 + Δx) -y (x 0) = y (4.01) -y (4) = 4.01 2 -4 2 = 16.0801-16 = 0.0801.
2. Find the angle of inclination of a tangent to a graph of a function y = f (x) at the point x 0, if f "(x 0) = 1.
Solution.
Derivative value at tangency point x 0 and there is the value of the tangent of the angle of inclination of the tangent (the geometric meaning of the derivative). We have: f "(x 0) = tanα = 1 → α = 45 °, because tg45 ° = 1.
Answer: the tangent to the graph of this function forms an angle with the positive direction of the Ox axis equal to 45 °.
3. Derive the formula for the derivative of a function y = x n.
Differentiation Is the action of finding the derivative of a function.
When finding derivatives, formulas are used that were derived based on the definition of the derivative, in the same way as we derived the formula for the derived degree: (x n) "= nx n-1.
These are the formulas.
Derivatives table it will be easier to memorize by pronouncing verbal formulations:
1. The derivative of a constant is zero.
2. The x stroke is equal to one.
3. The constant factor can be taken outside the sign of the derivative.
4. The derivative of the degree is equal to the product of the exponent of this degree by the degree with the same base, but the exponent is one less.
5. The derivative of a root is equal to one divided by two of the same roots.
6. The derivative of unit divided by x is equal to minus one divided by x squared.
7. The derivative of the sine is equal to the cosine.
8. The derivative of the cosine is equal to the minus sine.
9. The derivative of the tangent is equal to one divided by the square of the cosine.
10. The cotangent derivative is equal to minus one divided by the sine square.
We teach differentiation rules.
1.
The derivative of the algebraic sum is equal to the algebraic sum of the derivatives of the terms.
2. The derivative of the product is equal to the product of the derivative of the first factor by the second plus the product of the first factor by the derivative of the second.
3. The derivative of "y" divided by "ve" is equal to the fraction, in the numerator of which "y is the stroke multiplied by" ve "minus" y multiplied by the prime ", and in the denominator -" ve squared ".
4. A special case formulas 3.
We teach together!
Page 1 of 1 1
Edith Piaf: biography, best songs, interesting facts, listen to the story of Edith Piaf
Blackcurrant jelly
How many times a day should an adult pee - pee?