Consider a curvilinear trapezion bounded by the axis oh, the curve y \u003d f (x) and two straight: x \u003d a and x \u003d b (Fig. 85). Take the arbitrary value of x (only not and not b). We will give him the increment H \u003d DX and consider the strip bounded by straight AV and CD, the axis of OH and the Arc BD belonging to the curve under consideration. We will call this strip by an elementary strip. The area of \u200b\u200bthe elementary strip differs from the ACQB rectangle area to the curvilinear triangle BQD, and the area of \u200b\u200bthe latter is less than the BQDM rectangle area with the sides bq \u003d \u003d H \u003d Dx) QD \u003d AY and an area equal to Hay \u003d AY DX. With a decrease in the part H, the side of the remote control is also reduced and simultaneously with H striving for zero. Therefore, the BQDM area is an infinitely small second order. The area of \u200b\u200bthe elementary strip is the increment of the area, and the area of \u200b\u200bthe ACQB rectangle, equal to the AV-speech \u003d\u003d / (x) DX\u003e there is a differential area. Consequently, I will find the square itself by integrating its differential. Within the figure in question, the independent variable l: varies from a to b, so the desired area 5 will be 5 \u003d \\ f (x) dx. (I) Example 1. Calculate the area bounded by parabola in - 1 s *, straight x \u003d - fj-, x \u003d 1 and the axis of * (Fig. 86). In fig. 87. Fig. 86. 1 Here f (x) \u003d 1 - l?, The limits of integration A \u003d - and £ \u003d 1, therefore j [* -t] \\ - -fl - M -1- ± L_ 1V1 -LL-II- ^ 3) | _ 2 3V 2 / J 3 24 24 * Example 2. Calculate the area bounded by the sinusoid y \u003d sinxy axis oh and straight (Fig. 87). Using formula (I), we obtain l 2 s \u003d j sinxdx \u003d [-cos x] q \u003d 0 - (- 1) \u003d lf Example 3. Calculate the area, limited arc sinusoids ^ y \u003d sin jc, concluded between two adjacent intersection points With the axis oh (for example, between the beginning of the coordinates and the point with the abscissa I). Note that it is clear from the geometric considerations that this area will be twice as many areas of the previous example. However, we do computation: I'm 5 \u003d | S \\ NXDX \u003d [- COSH) * - - COS I - (- COS 0) \u003d 1 + 1 \u003d 2. Oh really, our assumption turned out to be fair. Example 4. Calculate the area bounded by the sinusoid and ^ axis oh on one ne rioode (Fig. 88). Preliminary raf rice suggests that the area will be four times more than in Ave. 2. However, making calculations, we get "I g, * I S - \\ Sin x DX \u003d [- COS x] 0 \u003d \u003d - COS 2L - (- COS 0) \u003d - 1 + 1 \u003d 0. This result requires clarification. To determine the essence of the case, we calculate the area bounded by the same sinusoid y \u003d sin l: and the axis oh, ranging from L to 2th. Using formula (I), we obtain 2 liters of $ 2l sin xdx \u003d [- cosx] l \u003d -cos 2, ~) -C05Y \u003d - 1-1 \u003d -2. In this way, we see that this area turned out to be negative. Comparing it with an area calculated in Ave. 3, we obtain that their absolute values \u200b\u200bare the same, and the signs are different. If you apply the V property (see ch. Xi, § 4), I received 2 liters 2l j sin xdx \u003d j sin * dx [sin x dx \u003d 2 + (- 2) \u003d 0, what happened in this example is not Accident. Always the area below the axis OH, provided that the independent variable changes from left to right, it turns out when calculating with the integrals of negative. In this course, we will always consider squares without signs. Therefore, the answer in just a disassembled example will be like this: the desired area is 2 + | -2 | \u003d 4. Example 5. Calculate the OAV area specified in Fig. 89. This area is limited to the axis oh, parabola y \u003d - xg and straight y - \u003d -x + \\. The area of \u200b\u200bthe curvilinear trapezium The desired area of \u200b\u200bthe OAV consists of two parts: OAM and MAV. Since point A is a point of intersection of parabola and straight, then its coordinates will find solving the system of equations 3 2 y \u003d TX. (We need to find only abscissa points a). Solving the system, we find l; \u003d ~. Therefore, the area has to be calculated in parts, first pl. OAM, and then pl. MAV: .... G 3 2, 3 g hp 3 1/2 in 2. QAM- ^ X and does not change his sign on it (Fig. 1).The area of \u200b\u200bthe curvilinear trapezium can be denoted by S (G).

A specific integral ʃ A B f (x) dx for the function f (x), which is continuous and non-negative on the segment [A; b], and there is an area of \u200b\u200bthe corresponding curvilinear trapezium.

That is, to find the area of \u200b\u200bthe figure G, limited by the lines y \u003d f (x), y \u003d 0, x \u003d a and x \u003d b, it is necessary to calculate a certain integral ʃ A B f (x) DX.

In this way, S (G) \u003d ʃ A b f (x) dx.

In case the function y \u003d f (x) is not positive on [A; b], then the area of \u200b\u200bthe curvilinear trapezium can be found by the formula S (G) \u003d -ʃ A B f (x) DX.

Example 1.

Calculate the area of \u200b\u200bthe figure bounded by the lines y \u003d x 3; y \u003d 1; x \u003d 2.

Decision.

The specified lines form an ABC figure, which is shown by hatching on fig. 2.

The desired area is equal to the difference between the DACE curvilinear trapezium areas and the Dabe square.

Using the formula S \u003d ʃ A B f (x) dx \u003d s (b) - s (a), we will find the integration limits. To do this, solve the system of two equations:

(y \u003d x 3,
(y \u003d 1.

Thus, we have x 1 \u003d 1 - the lower limit and x \u003d 2 - the upper limit.

So, S \u003d S DACE - S Dabe \u003d ʃ 1 2 x 3 dx - 1 \u003d x 4/4 | 1 2 - 1 \u003d (16 - 1) / 4 - 1 \u003d 11/4 (sq.).

Answer: 11/4 kV. units.

Example 2.

Calculate the area of \u200b\u200bthe figure limited by the lines y \u003d √h; y \u003d 2; x \u003d 9.

Decision.

The specified lines form a figure of ABC, which is limited from above the graph

y \u003d √h, and below the graph of the function y \u003d 2. The resulting figure is shown by hatching on fig. 3.

The desired area is S \u003d ʃ A B (√X - 2). We will find the integration limits: B \u003d 9, for finding a, by solving the system of two equations:

(y \u003d √h,
(y \u003d 2.

Thus, we have that x \u003d 4 \u003d a is the lower limit.

So, S \u003d ∫ 4 9 (√X - 2) DX \u003d ∫ 4 9 √X DX -∫ 4 9 2DX \u003d 2/3 x√ x | 4 9 - 2x | 4 9 \u003d (18 - 16/3) - (18 - 8) \u003d 2 2/3 (sq.).

Answer: S \u003d 2 2/3 square meters. units.

Example 3.

Calculate the area of \u200b\u200bthe figure, limited by the lines y \u003d x 3 - 4x; y \u003d 0; x ≥ 0.

Decision.

We construct a graph of the function y \u003d x 3 - 4x at x ≥ 0. To do this, find the derivative of the ':

y '\u003d 3x 2 - 4, y' \u003d 0 at x \u003d ± 2 / √3 ≈ 1.1 - critical points.

If you depict critical points on the numeric axis and set the signs of the derivative, we obtain that the function decreases from zero to 2 / √3 and increases from 2 / √3 to plus infinity. Then x \u003d 2 / √3 is a minimum point, the minimum value of the function in MIN \u003d -16 / (3√3) ≈ -3.

We define the intersection points of the graph with the axes of coordinates:

if x \u003d 0, then y \u003d 0, and therefore, and (0; 0) - the intersection point with the OU axis;

if y \u003d 0, then x 3 - 4x \u003d 0 or x (x 2 - 4) \u003d 0, or x (x - 2) (x + 2) \u003d 0, where x 1 \u003d 0, x 2 \u003d 2, x 3 \u003d -2 (not suitable, because x ≥ 0).

Points A (0; 0) and in (2; 0) - the intersection points of the graph with the axis oh.

The specified lines form a figure of the OAV, which is shown by hatching on fig. four.

Since the function y \u003d x 3 - 4x takes on (0; 2) a negative value, then

S \u003d | ʃ 0 2 (x 3 - 4x) DX |.

We have: ʃ 0 2 (x 3 - 4x) dx \u003d (x 4/4 - 4x 2/2) | 0 2 \u003d -4, from where s \u003d 4 square meters. units.

Answer: S \u003d 4 square meters. units.

Example 4.

Find the area of \u200b\u200bthe figure limited by parabola y \u003d 2x 2 - 2x + 1, straight x \u003d 0, y \u003d 0 and tangent of this parabole at the point with the abscissa x 0 \u003d 2.

Decision.

First, the equation is tangent to parabole y \u003d 2x 2 - 2x + 1 at the point with the abscissa x₀ \u003d 2.

Since the derivative y '\u003d 4x - 2, then at x 0 \u003d 2, we obtain k \u003d y' (2) \u003d 6.

We find the ordinate point of touch: at 0 \u003d 2 · 2 2 - 2 · 2 + 1 \u003d 5.

Consequently, the equation of tangent has the form: y - 5 \u003d 6 (x - 2) or y \u003d 6x - 7.

Build a figure limited lines:

y \u003d 2x 2 - 2x + 1, y \u003d 0, x \u003d 0, y \u003d 6x - 7.

G y \u003d 2x 2 - 2x + 1 - parabola. Intersection points with coordinate axes: A (0; 1) - with OU axis; with the axis oh - there are no points of intersection, because Equation 2x 2 - 2x + 1 \u003d 0 does not have solutions (D< 0). Найдем вершину параболы:

x b \u003d 2/4 \u003d 1/2;

y B \u003d 1/2, that is, the vertex parabola point B has coordinates in (1/2; 1/2).

So, the figure whose area is required to determine is shown by hatching on fig. five.

We have: S O A in D \u003d S OABC - S ADBC.

We will find the coordinates of the point D from the condition:

6x - 7 \u003d 0, i.e. x \u003d 7/6, it means Dc \u003d 2 - 7/6 \u003d 5/6.

DBC triangle area Find according to the formula S ADBC \u200b\u200b\u003d 1/2 · DC · BC. In this way,

S adbc \u003d 1/2 · 5/6 · 5 \u003d 25/12 kV. units.

S OABC \u003d ʃ 0 2 (2x 2 - 2x + 1) DX \u003d (2x 3/3 - 2x 2/2 + x) | 0 2 \u003d 10/3 (sq. Food.).

We finally obtain: S O A in D \u003d S OABC - S ADBC \u200b\u200b\u003d 10/3 - 25/12 \u003d 5/4 \u003d 1 1/4 (sq. M. Uzh).

Answer: S \u003d 1 1/4 kV. units.

We disassembled examples finding the squares of figures limited by the specified lines. To successfully solve such tasks, you must be able to build on the plane of the line and graphs of functions, find the points of intersection of lines, apply the formula for finding the area, which implies the presence of skills and skills for calculating certain integrals.

the site, with full or partial copying of the material reference to the original source is required.

Any particular integral (which exists) has a very good geometric meaning. At the lesson, I said that a certain integral is a number. And now it's time to state another useful fact. From the point of view of geometry, a certain integral is an area.

I.e, a specific integral (if it exists) geometrically corresponds to the area of \u200b\u200bsome figure. For example, consider a specific integral. The integrand function sets some curve on the plane (it can always be drawn if desired), and a certain integral itself is numerically equal to the area of \u200b\u200bthe corresponding curvilinear trapezium.

Example 1.

This is a typical task formulation. The first and most important point of the decision - building a drawing. And the drawing must be built RIGHT.

When building a drawing, I recommend the following order: first it is better to build all straight (if they are) and only later - Parabolas, hyperbolas, schedules of other functions. Function graphs are more profitable to build potochoeThe technique of check-in construction can be found in the reference material.

There you can also find a very useful material in relation to our lesson the material - how to quickly build a parabola.

In this task, the decision may look like that.
Perform the drawing (note that the equation sets the axis):

I will not stroke a curvilinear trapeze, it is obvious here about which area there is a speech. The decision continues like this:

On the segment schedule a function is located over the axis, so:

Answer:

Who has difficulties with the calculation of a certain integral and the use of Newton-Leibnia formula , refer to the lecture Certain integral. Examples of solutions.

After the task is completed, it is always useful to look at the drawing and estimate, the real one turned out. In this case, "on the eyes" we count the number of cells in the drawing - well, approximately 9 will be flown, it seems to the truth. It is quite clear that if we had, say, answer: 20 square units, it is obvious that an error is made somewhere - in the figure of 20 cells, it is clearly not fitted, from the strength of a dozen. If the answer turned out negative, the task is also decided incorrectly.

Example 2.

Calculate the area of \u200b\u200bthe shape, limited lines, and axis

This is an example for an independent solution. Complete solution and answer at the end of the lesson.

What to do if the curvilinear trapezium is located under the axis?

Example 3.

Calculate the area of \u200b\u200bthe shape, limited lines, and the coordinate axes.

Solution: Perform drawing:

If a curvilinear trapezium fully located under the axis, then its area can be found by the formula:
In this case:

Attention! Do not confuse two types of tasks:

1) If you are invited to solve a simple integral without any geometric meaning, then it may be negative.

2) If you are invited to find the figure of the figure using a specific integral, then the area is always positive! That is why in just the considered formula appears minus.

In practice, the figure is most often located in the upper and lower half plane, and therefore, from the simplest school charts, go to more meaningful examples.

Example 4.

Find the area of \u200b\u200ba flat figure, limited lines ,.

Solution: First you need to draw a drawing. Generally speaking, when building a drawing in tasks to the area, we are most interested in the intersection points of the lines. Find points of intersection of parabola and direct. This can be done in two ways. The first method is analytical. We solve the equation:

So, the lower integration limit, the upper limit of integration.
This way is better, if possible, do not use.

It is much more profitable and faster to build the lines of the line, while the integration limits are clarified as if "by themselves". The technique of the cessation for various graphs is considered in detail in the help Charts and properties of elementary functions. However, an analytical way to find the limits after all, it is sometimes necessary to apply if, for example, the schedule is large enough, or a trained construction did not reveal the integration limits (they can be fractional or irrational). And such an example, we also consider.

We return to our task: more rational first build a straight line and only then Parabola. Perform drawing:

I repeat that in the current construction, the integration limits are most often found out by the "automatic".

And now the working formula: If on the segment some continuous function more or equal Some continuous function, the area of \u200b\u200bthe corresponding figure can be found by the formula:

Here it is no longer necessary to think where the figure is located - over the axis or under the axis, and, roughly speaking, important what is the graph above(relative to another schedule) and what - below.

In this example, it is obvious that on the segment of Parabola is located above straight, and therefore it is necessary to subtract

Completion of the solution may look like this:

The desired figure is limited to parabola from above and direct bottom.
On the segment, according to the corresponding formula:

Answer:

In fact, the school formula for the area of \u200b\u200bthe curvilinear trapezium in the lower half-plane (see simple example No. 3) - a special case of formula . Since the axis is defined by the equation, and the function graph is located below the axis,

And now a couple of examples for an independent decision

Example 5.

Example 6.

Find the area of \u200b\u200bthe figure limited lines ,.

In the course of solving tasks for calculating the area with a specific integral, a funny case occurs sometimes. The drawing is completed correctly, calculations - right, but intensified ... found the area is not the figureThat this is how your humble servant was packed. Here is a real case from life:

Example 7.

Calculate the area of \u200b\u200bthe shape, limited lines ,,,.

First execute the drawing:

Figure whose area we need to find is shaded in blue(Look carefully on the condition - than the figure is limited!). But in practice on inattention, it is often that it is necessary to find the area of \u200b\u200bthe figure, which is shaded with green!

This example is also useful in that it is considered to be in it the size of two specific integrals. Really:

1) A straight schedule is located on the segment over the axis;

2) On the segment over the axis there is a graph of hyperboles.

It is clear that the square can (and need) to decompose, so:

Answer:

Example 8.

Calculate the area of \u200b\u200bthe shape, limited lines,
Imagine the equation in the "school" form, and perform the current drawing:

From the drawing it is clear that the upper limit we have "good" :.
But what is the lower limit?! It is clear that this is not an integer, but what? May be ? But where is the guarantee that the drawing is made with perfect accuracy, it may well be that. Or root. And if we generally improperly built a schedule?

In such cases, you have to spend extra time and specify the integration limits analytically.

Find the intersection points of the direct and parabola.
To do this, solve the equation:

Hence, .

Further solution is trivial, the main thing is not to get confused in substitutions and signs, the calculations here are not the simplest.

On cut According to the corresponding formula:

Answer:

Well, and in the conclusion of the lesson, consider two tasks more difficult.

Example 9.

Calculate the area of \u200b\u200bthe shape, limited lines ,,

Solution: Show this shape in the drawing.

For the current construction of the drawing, it is necessary to know the appearance of the sinusoids (and it is generally useful to know graphs of all elementary functions), as well as some sinus values, they can be found in trigonometric table. In some cases (as in this), it is allowed to build a schematic drawing on which the graphs and integration limits must be reflected in principle.

With the limits of integration, there are no problems here, they follow directly from the condition: - "X" varies from zero to "pi". We draw up a further solution:

On the segment, the function graph is located above the axis, so:

(1) How to integrate sinuses and cosines in odd degrees can be viewed at the lesson Integrals from trigonometric functions. This is a typical reception, pressing one sinus.

(2) We use the main trigonometric identity in the form of

(3) We will replace the variable, then:

New alteration integration:

Who has very bad things with replacements, please go to the lesson Replacement method in an indefinite integral. Who is not very clear to the replacement algorithm in a specific integral, visit the page Certain integral. Examples of solutions.

Introduction

Finding a derivative F "(x) or differential DF \u003d F" (x) DX Function F (X) is the main task of differential calculus. In the integral calculation, the inverse problem is solved: according to a given function f (x) it is required to find such a function f (x), which f "(x) \u003d f (x) or f (x) \u003d f" (x) dx \u003d f (x ) DX. Thus, the main task of integral calculus is the restoration of the function f (x) according to the known derivative (differential) of this function. Integrative calculation has numerous applications in geometry, mechanics, physics and technology. It gives a general method of finding space, volumes, centers of gravity, etc.

The course of mathematical analysis contains a variety of material, however, one of its central partitions is a specific integral. The integration of many types of functions is sometimes one of the most difficult problems of mathematical analysis.

Calculation of a specific integral has not only theoretical interest. It is sometimes reduced to its calculation, the tasks associated with the practical activities of a person are reduced.

Also, the concept of a specific integral is widely used in physics.

Finding the area of \u200b\u200bthe curvilinear trapezium

A curvilinear trapezium is called a figure located in a rectangular coordinate system and a limited abscissa axis, direct x \u003d A. and x \u003d B. and the curve, and non-negative on the segment. Approximately the area of \u200b\u200bthe curvilinear trapezium can be found like this:

1. To divide the cut of the abscissa axis on n. equal segments;

2. Conduct the sections of the segments perpendicular to the abscissa axis, to the intersection with the curve;

3. Replace the resulting rectangle columns with a base and a height equal to the value of the function f. in the left end of each segment;

4. Find the sum of the squares of these rectangles.

But you can find the area of \u200b\u200bcurvilinear otherwise: according to the formula Newton labitsa. To prove the formula that begins, we prove that the area of \u200b\u200bthe curvilinear trapezium is equal to, where - any of the primitive functions whose graph limits the curvilinear trapezium.

The calculation of the area of \u200b\u200bthe curvilinear trapezium is written as follows:

1. There is any of the primitive functions.

2. Recorded. - This is a Newton-Leibniz formula.

Finding the area of \u200b\u200bthe curvilinear sector

Consider the curve? \u003d? (?) In the polar coordinate system, where? (?) - continuous and non-negative on [?; ?] function. Figure, limited curve? (?) and rays? \u003d?,? \u003d?, is called the curvilinear sector. The area of \u200b\u200bthe curvilinear sector is equal to

Finding the length of the arc curve

Rectangular coordinates

Suppose in rectangular coordinates a flat curve AB is given, the equation of which y \u003d f (x), where a? x? b. (Figure 2)

Under the arc length, AB is understood as the limit to which the length of the broken line, inscribed in this arc, when the number of links of the broken is increasingly increasing, and the length of the greatest link should strive for zero.

Apply scheme I (summation).

Points x \u003d a, x, ..., x \u003d b (x? X? X \u003d b (x? X? X) Severe the segment on N parts. Let these points correspond to the points M \u003d A, M, ..., M \u003d B on the AB curve. We will conduct chords MM, MM, ..., MM, whose lengths are denoted by respectively through? L ,? L, ... ,? L.

We obtain broken MMM ... mm, the length of which is L \u003d? L +? L + ... +? L \u003d? L.

The length of the chord (or Lymannaya link)? L can be found on the Pythagoree theorem from a triangle with customs? X and? Y:

L \u003d, where? X \u003d x - x ,? y \u003d f (x) - f (x).

According to the Lagrange Theorem on the final increment of the function

Y \u003d (c)? X, where c (x, x).

and the length of all broken MMM ... mm is equal

The length of the AB curve, by definition, is equal

Note that when? L 0 also and? X 0 (? L \u003d and therefore |? x |< ?L). Функция непрерывна на отрезке , так как, по условию, непрерывна функция f (X). Следовательно, существует предел интегральной суммы L=?L= , кода max ?X 0:

Thus, L \u003d DX.

Example: Find the length of the circle of the radius R. (Fig. 3)

Find? Part of its length from the point (0; r) to the point (R; 0). As

№ ____ Date ________

Subject:Curvilinear trapezium and its square b

Objectives lesson: Give the definition of a curvilinear trapezium and its square, learn to calculate the area of \u200b\u200bthe curvilinear trapezium.

DURING THE CLASSES

1. Organizational moment.

Greeting students, checking class readiness for lesson, organization of student attention, disclosure of the general objectives of the lesson and its plan.

2. Stage checking homework.

Objectives: establish the correctness, completeness and awareness of the implementation of d / s all students, identify gaps in knowledge and ways of students' activity. Determine the causes of the occurrence of difficulties, eliminate the discovered spaces.

3. Top update.

Tasks: Providing the motivation of schoolchildren's teachings, inclusion in joint activities to determine the objectives of the lesson. Actualize subjective student experience.

Recall the basic concepts and formulas.

Definition. Function y \u003d.f.(x), x (A, B), called primitive for function y \u003d f (x), x (A, B), If for everyone x. (A, B) Equality is performed

F. (x) \u003d f (x).

Comment. If a f.(x) there is a primitive for function f (X), then with any constant FROM, F (x) + C is also a primitive for f (x).

The task of finding all the primitive functions f (X) called integration, and the set of all the primary is called an uncertain integral for the function f (X) by DX And denotes

Properties include:

1. ;

2. If a C \u003d.Const, T.
;

3.
.

Comment. In the school course of mathematics, the term "indefinite integral" is not used, instead they say "Many of all the primordial".

We give a table of uncertain integrals.

Example 1. Find a primary for function
passing through the point M.(2;4).

Decision. Many of all primitive functions
There is an indefinite integral
. I calculate it using the properties of the integral 1 and 2. We have:

Received that the set of all the primitive is given by the family of functions y \u003d F (x) + C, i.e y \u003d X. 3 – 2x + C.where FROM - Arbitrary constant.

Knowing that the primary pass through the point M.(2; 4), we will substitute its coordinates in the previous expression and find FROM.

4=2 3 –2 2+FROM  FROM=4–8+4; FROM=0.

Answer: F (x) \u003d x 3 - 2x. - the desired primitive.

4. Formation of new concepts and methods of action.

Tasks: Ensure perception, understanding and memorizing student under the material under study. Ensure the learning methodology for the reproduction of the studied material, promote the philosophical understanding of the appropriate concepts, laws, rules, formulas. To establish the correctness and awareness of the studied material learned, to identify the gaps of primary comprehension, conduct correction. Provide correlated by students of its subjective experience with signs of scientific knowledge.

Finding faces of flat figures

The task of finding an area of \u200b\u200ba flat figure is closely related to the task of finding primitive (integration). Namely: the area of \u200b\u200bthe curvilinear trapezium limited graphy \u003d f (x) (f (x)\u003e 0) straightx \u003d a; x \u003d b; y \u003d. 0, equal to the difference difference values \u200b\u200bfor the functiony \u003d f (x) At pointsb. anda. :

S \u003d F (B) -F (A)

Let us give the definition of a specific integral.

ABOUT
ratio. Let the function y \u003d f (x) Defined and integrable on the segment [ a, B.] let it go F (X) - Some of her primitive. Then the number F (b) -f (a) called integral OT. but before b. Functions f (X) And denotes

.

Equality
called Newton Labitsa Formula.

This formula connects the task of finding a flat figure with an integral.

In general, if the figure is limited to graphs of functions y \u003d f (x); Y \u003d G (x) (f (x)\u003e g (x)) And straight x \u003d A.; X \u003d B.then its area is equal:

.

Example2. At what point is the function schedule y \u003d X. 2 + 1 It is necessary to hold a tangent, so that it cuts out from the figure formed by the schedule of this function and straight y \u003d.0, x \u003d0, x \u003d1 trapezing the largest square?

Decision. Let be M. 0 (X. 0 , y. 0 ) - function graphics function y \u003d X. 2 + 1, in which the desired tangent.

Find the equation tangent y \u003d y. 0 + F. (X. 0 ) (X-X 0 ) .

We have:

therefore

.

Find the square of the trapezium OAUKS.

.

B. - point of intersection of tangent with direct x \u003d.1 

The task is reduced to finding the greatest value of the function.

S.(x.)\u003d -X. 2 + X +.1 on the segment. Find S. (x.)=– 2x +.1. Find a critical point from the condition S. (x.)= 0  x \u003d..

We see that the function reaches the greatest value when x \u003d.. Find
.

Answer: tangentic need to spend at point
.

Note that the task of finding the integral is often found, based on its geometrical meaning. Let us show on the example how such a task is solved.

Example 4. Using the geometric meaning of the integral to calculate

but )
; b)
.

Decision.

but)
- equal to the area of \u200b\u200bcurvilinear trapezium, limited lines.

P reform

- Upper half of the circle with the center R(1; 0) and radius R \u003d.1.

therefore
.

Answer: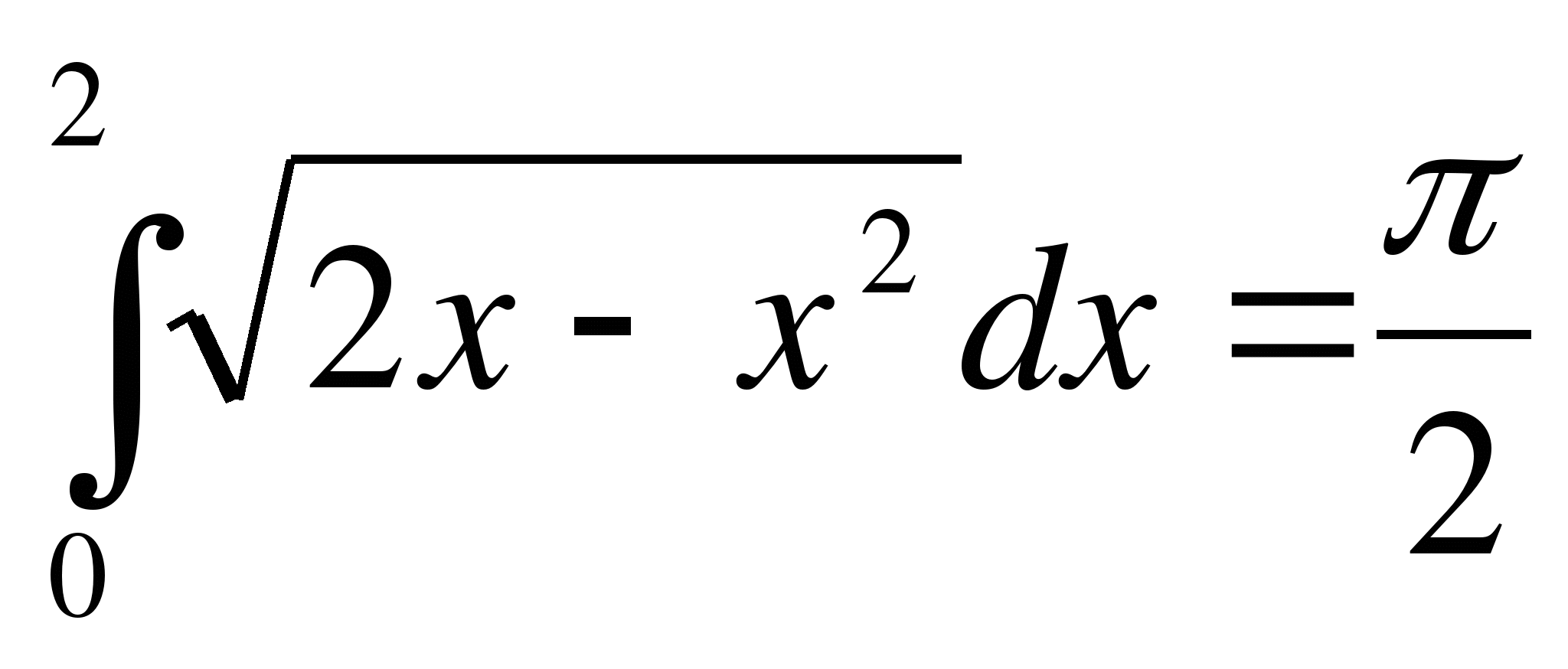
.

b) arguing similarly, we will build a region limited by charts .2 – 2x +.2, tangent to it at points A.
, B.(4;2)

y \u003d.–9x-59, parabola y \u003d.3x. 2 + AX +.1, if you know that tangent to Parabola at the point x \u003d -2 is with the axis OX. The angle of magnitude arctg.6.

To find butif it is known that the area of \u200b\u200bthe curvilinear trapezium limited y \u003d.3x. 3 + 2x, x \u003d a, y \u003d0, equal to one.

Find the smallest value of the area of \u200b\u200bthe figure limited by parabola y \u003d X. 2 + 2x-3 and direct y \u003d kx +1.

6.Tap information about your homework.

Tasks: to ensure an understanding of the goals, content and methods of homework.№18, 19,20,21 odd

7.Instation of the lesson.

Task: give a qualitative assessment of class work and individual students.